Control Systems is a fantastic subject and I wanted to write some topics regarding that. The first thing you notice in control system class is to find the gain of a "PRETTY BIG BLOCK" by using various reductions techniques. The steps involved will be :
1) Multiply the gain(G) of the blocks which are in series and add the gains(G) which are in parallel
2) Switch the take-off point to the right side of the block and blah-blah stuff.
So, in order to avoid all these steps, one brilliant lad, invented a formula, which is named after him(We are accustomed to FORMULA, isn't it? ). That formula makes life easy for us who study control system. That formula is called, MASON'S GAIN FORMULA. Mason's gain formula applies only to LINEAR SYSTEMS.
If you don't know what Linear System is? Google has a quick ans : A general deterministic system can be described by operator,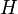 
, that maps an input, 
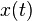, as a function of 
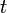to an output, 
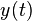, a type of black box description. Linear systems satisfy the properties of superposition and scaling or homogeneity.
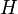, that maps an input, 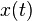, as a function of 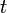to an output, 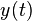, a type of black box description. Linear systems satisfy the properties of superposition and scaling or homogeneity.
Mason's gain formula Example.
I have solved a Signal Flow graph using Mason's gain formula. The explanation would be suffice I guess. Kindly go through the snapshots and revert back for any doubts.
Tip:Zoom the pic for better clarity
